Proposition 13

Let ABCDE be the given equilateral and equiangular pentagon.

It is required to inscribe a circle in the pentagon ABCDE.

Bisect the angles BCD and CDE by the straight lines CF and DF respectively. Join the straight lines FB, FA, and FE from the point F at which the straight lines CF and DF meet one another.

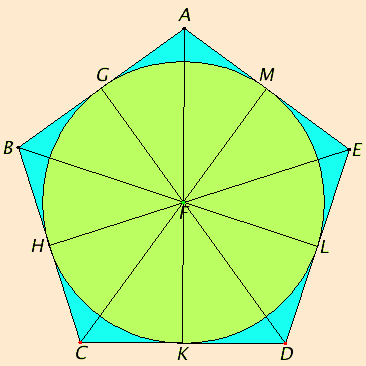
Then, since BC equals CD, and CF common, the two sides BC and CF equal the two sides DC and CF, and the angle BCF equals the angle DCF, therefore the base BF equals the base DF, and the triangle BCF equals the triangle DCF, and the remaining angles equal the remaining angles, namely those opposite the equal sides.

Therefore the angle CBF equals the angle CDF.

And, since the angle CDE is double the angle CDF, and the angle CDE equals the angle ABC, while the angle CDF equals the angle CBF, therefore the angle CBA is also double the angle CBF. Therefore the angle ABF equals the angle FBC. Therefore the angle ABC is bisected by the straight line BF.

Similarly it can be proved that the angles BAE and AED are also bisected by the straight lines FA and FE respectively.

Now draw FG, FH, FK, FL, and FM from the point F perpendicular to the straight lines AB, BC, CD, DE, and EA.

Then, since the angle HCF equals the angle KCF, and the right angle FHC also equals the angle FKC, FHC and FKC are two triangles having two angles equal to two angles and one side equal to one side, namely FC which is common to them and opposite one of the equal angles, therefore they also have the remaining sides equal to the remaining sides. Therefore the perpendicular FH equals the perpendicular FK.

Similarly it can be proved that each of the straight lines FL, FM, and FG also equals each of the straight lines FH and FK, therefore the five straight lines FG, FH, FK, FL, and FM equal one another.

Therefore the circle described with center F and radius one of the straight lines FG, FH, FK, FL, or FM also passes through the remaining points, and it touches the straight lines AB, BC, CD, DE, and EA, because the angles at the points G, H, K, L, and M are right.

For, if it does not touch them. but cuts them, it will result that the straight line drawn at right angles to the diameter of the circle from its end falls within the circle, which was proved absurd.

Therefore the circle described with center F and radius one of the straight lines FG, FH, FK, FL, or FM does not cut the straight lines AB, BC, CD, DE, and EA. Therefore it touches them.

Let it be described, as GHKLM.

Therefore a circle has been inscribed in the given equilateral and equiangular pentagon.