Proposition 6
Let ABCD be the given circle.
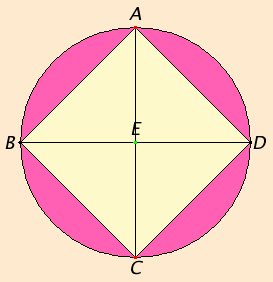
It is required to inscribe a square in the circle ABCD.
Draw two diameters AC and BD of the circle ABCD at right angles to one another, and join AB, BC, CD, and DA.
Then, since BE equals ED, for E is the center, and EA is common and at right angles, therefore the base AB equals the base AD.
For the same reason each of the straight lines BC and CD also equals each of the straight lines AB and AD. Therefore the quadrilateral ABCD is equilateral.
I say next that it is also right-angled.
For, since the straight line BD is a diameter of the circle ABCD, therefore BAD is a semicircle, therefore the angle BAD is right.
For the same reason each of the angles ABC, BCD, and CDA is also right. Therefore the quadrilateral ABCD is right-angled.
But it was also proved equilateral, therefore it is a square, and it has been inscribed in the circle ABCD.
Therefore the square ABCD has been inscribed in the given circle.
