Proposition 7
Let ABCD be the given circle.
It is required to circumscribe a square about the circle ABCD.
Draw two diameters AC and BD of the circle ABCD at right angles to one another.
Draw FG, GH, HK, and KF through the points A, B, C, and D touching the circle ABCD.
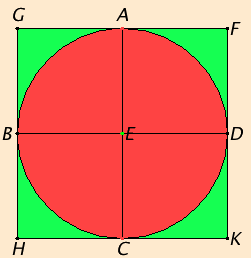
Then, since FG touches the circle ABCD, and EA has been joined from the center E to the point of contact at A, therefore the angles at A are right. For the same reason the angles at the points B, C, and D are also right.
Now, since the angle AEB is right, and the angle EBG is also right, therefore GH is parallel to AC.
For the same reason AC is also parallel to FK, so that GH is also parallel to FK.
Similarly we can prove that each of the straight lines GF and HK is parallel to BED.
Therefore GK, GC, AK, FB, and BK are parallelograms, therefore GF equals HK, and GH equals FK.
And, since AC equals BD, and AC also equals each of the straight lines GH and FK, and BD equals each of the straight lines GF and HK, therefore the quadrilateral FGHK is equilateral.
I say next that it is also right-angled.
For, since GBEA is a parallelogram, and the angle AEB is right, therefore the angle AGB is also right.
Similarly we can prove that the angles at H, K, and F are also right. Therefore FGHK is right-angled.
But it was also proved equilateral, therefore it is a square, and it has been circumscribed about the circle ABCD.
Therefore a square has been circumscribed about the given circle.
